Solved Questions on Area of Polygons
1. Find the area of a regular hexagon with a perimeter of 36 cm.
a) 93.53 cm2
b) 98.23 cm2
c) 86.28 cm2
d) 66.66 cm2
Answer: a) 93.53 cm2
Explanation: Since a hexagon has six sides, the length of each side is 36 cm / 6 = 6 cm.
Using the formula for the area of a regular polygon, we have: A = (3√3 / 2) x a2
= (3√3 / 2) x 62
= (3√3 / 2) x 36
= 93.53 cm2
2. A rhombus has diagonals of lengths 6 cm and 8 cm. What is the area of the rhombus?
a) 12 cm²
b) 24 cm²
c) 30 cm²
d) 48 cm²
Answer: b)
Explanation: The area of a rhombus = (d1 x d2) / 2
where d1 and d2 are the lengths of the diagonals.
Plugging in the given values, we get:
Area = (6 x 8) / 2
Area = 24 cm²
Therefore, the answer is (b) 24 cm².
3. Find the area of the figure given below:
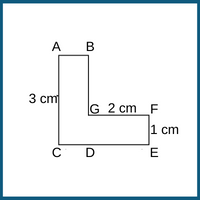
a) 5 cm2
b) 6 cm2
c) 7 cm2
d) 8 cm2
Answer: a) 5 cm2
Explanation: Split the above diagram into two rectangles
Area of a rectangle ABDC = 3 × 1 = 3 cm2
Area of a rectangle EFGD = 2 × 1 = 2 cm2
Therefore, Total Area = Area of a rectangle ABDC + Area of a rectangle EFGD
= 3 + 2 cm2 = 5 cm2
Area of the given figure = 5 cm2
4. A parallelogram has a base of 6 cm and a height of 8 cm. What is the area of the parallelogram?
a) 12 cm²
b) 24 cm²
c) 32 cm²
d) 48 cm²
Answer: d) 48 cm²
Explanation: The formula for the area of a parallelogram is base times height. Using the given values, we have:
Area = 6 cm × 8 cm = 48 cm²
5. A rectangular garden measures 10 m by 15 m. What is the area of the garden?
a. 100 m2
b. 150 m2
c. 200 m2
d. 250 m2
Answer: b) 150 m2
Explanation: Area = 10 m x 15 m = 150 m2
Therefore, the area of the garden is 150 m2.
Practice Questions on Area of Polygon
1. A rhombus has diagonals of lengths 10 cm and 12 cm. What is the area of the rhombus?
a) 60 cm²
b) 120 cm²
c) 144 cm²
d) 240 cm²
Answer: a) 60 cm²
2. A triangle has a base of 8 cm and a height of 12 cm. What is the area of the triangle in cm2?
a) 32 cm2
b) 36 cm2
c) 48 cm2
d) 52 cm2
Answer: c) 48 cm2
3. A trapezium has a height of 6 m, a top base of 8 m, and a bottom base of 12 m. What is the area of the trapezium in m2?
a) 40 m2
b) 52 m2
c) 58 m2
d) 60 m2
Answer: d) 60 m2
4. Find the area of an equilateral triangle in cm2 with a side length 4 cm.
a) 4√3
b) 5√2
c) 7√3
d) 12√3
Answer: a) 4√3
5. A regular hexagon has a side length of 6 m. What is the area of the hexagon?
a) 24√3 m2
b) 72√3 m2
c) 60√3 m2
d) 54√3 m2
Answer: d) 54√3 m2
6. A square has an area of 144 square feet. What is the length of each side of the square?
a) 12 feet
b) 16 feet
c) 18 feet
d) 24 feet
Answer: a) 12 feet
7. The side of an equilateral triangle of area 64√3 cm2 is:
a) 12 cm
b) 14 cm
c) 16 cm
d) 18 cm
Answer: c) 16 cm
8. Find the area of the figure given below:
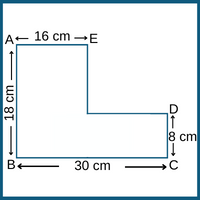
a) 200 cm2
b) 400 cm2
c) 600 cm2
d) 800 cm2
Answer: b) 400 cm2
9. Find the area of the figure given below:
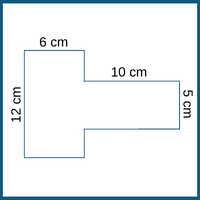
a) 156 cm2
b) 132 cm2
c) 122 cm2
d) 100 cm2
Answer: c) 122 cm2
10. If the area of a parallelogram is 24 cm² and its height is 4 cm, what is the length of its base?
a) 2 cm
b) 4 cm
c) 6 cm
d) 8 cm
Answer: c) 6 cm




