1. Among the following diagrams, select the one that inaccurately represents the refraction of a ray of light through a convex lens.
a) 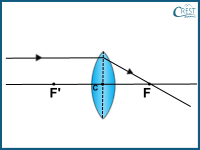
b) 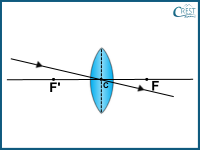
c) 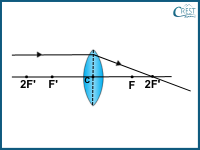
d) 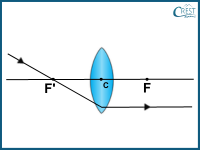
Answer: c) Ray diagram in option c inaccurately represents the refraction of a ray of light through a convex lens because it shows the ray of light, which is parallel to the principal axis of the lens, passing through 2F after refraction. This depiction is incorrect because, in reality, parallel rays of light passing through a convex lens converge to a single focal point (F), not 2F.
2. A wildlife photographer is using a telephoto lens with a focal length of 150 mm to capture an image of a fast-moving bird that is 10 cm tall. If the bird is located 50 cm in front of the lens, what is the distance at which the film should be placed to obtain a clear image of the bird in flight? Additionally, what will be the exact height of the bird's image on the film?
a) Film should be placed at 12.5 cm, and image height is 3.57 cm.
b) Film should be placed at 21.4 cm, and image height is 4.2 cm.
c) Film should be placed at 25.6 cm, and image height is 5 cm.
d) Film should be placed at 15 cm, and image height is 3 cm.
Answer: b) To determine the distance at which the film should be placed to obtain a clear image of the bird in flight and the height of the image of the bird on the film, we can use the lens formula: 1/f = 1/v - 1/u
Where:
f = 150 mm = 15 cm (focal length of the lens)
u = -50 cm (object distance, negative sign because it is positioned to the left of the lens)
v =? (image distance)
1/15 = 1/v - 1/-50
1/15 = 1/v + 1/50
1/v = 1/15 - 1/50
1/v = 7/150
v = 150/7 cm ≈ 21.4 cm
To calculate the height of the image (h2) on the film:
We have the equation for magnification: m = h2/h1 = v/u
h2 (height of the image) = ?
h1 (height of the bird) = 10 cm
v (image distance) = 21.4 cm
u (object distance) = 50 cm
Solving for h2:
h2/10 = 21.4/50
h2 ≈ 4.2 cm
So, the distance at which the film should be placed to obtain a clear image of the bird in flight is approximately 21.4 cm, and the height of the image of the bird on the film is approximately 4.2 cm.
3. If medium X has a refractive index of 3/2 with respect to air, and medium Y has a refractive index of 2/3 with respect to air, what is the refractive index of medium X with respect to medium Y?
a) 1
b) 1.25
c) 0.45
d) 2.25
Answer: d) To find the refractive index of medium X with respect to medium Y, you can use the relationship between the refractive indices of three media:
Refractive index of medium X with respect to medium Y (X/Y) = Refractive index of medium X with respect to air (X/air) divided by the refractive index of medium Y with respect to air (Y/air).
Given:
Refractive index of medium X with respect to air (X/air) = 3/2
Refractive index of medium Y with respect to air (Y/air) = 2/3
Now, calculate the refractive index of medium X with respect to medium Y: (X/Y) = (X/air) / (Y/air) = (3/2) / (2/3)
To divide fractions, multiply the first fraction by the reciprocal of the second fraction: (X/Y) = (3/2) x (3/2) = 9/4 = 2.25
So, the refractive index of medium X with respect to medium Y is 2.25.
4. A prescription lens has a power of -3.00 D.
I. Is this lens concave or convex?
II. Calculate the focal length of the lens
a) I: Concave; II: -0.33 metres.
b) I: Convex; II: 0.33 metres.
c) I: Concave; II: 0.33 metres
d) I: Convex; II: -0.33 metres
Answer: a) In eyeglass prescriptions, a negative power indicates a concave (diverging) lens. So, the lens with a power of -3.00 D is a concave lens.
To calculate the focal length (f) of a lens from its power (P) in diopters, you can use the formula:
f = 1 / P
In this case, P = -3.00 D, so:
f = 1 / (-3.00 D)
f = -1/3 metres (since 1 D = 1 m-1)
The negative sign indicates that the focal length is negative, which is characteristic of a concave lens. Therefore, the focal length of this lens is -1/3 metres or approximately -33.33 centimetres or -0.33 metres.
5. When an oblique ray of light passes from a rarer medium into an optically denser medium, what can be said about the angle of refraction compared to the angle of incidence?
a) The angle of refraction is always greater than the angle of incidence.
b) The angle of refraction is always less than the angle of incidence.
c) The angle of refraction is equal to the angle of incidence.
d) The angle of refraction depends on the colour of light.
Answer: b) As the ray of light transitions from the rarer medium to the denser medium, the leading edge of the ray enters the denser medium first and slows down, while the rest of the ray in the rarer medium continues at its initial speed. This speed difference between the leading edge and the rest of the ray causes the direction of the ray to bend toward the normal (an imaginary line perpendicular to the surface) as it enters the denser medium.
Since the entire ray eventually enters the denser medium and continues to bend, the angle of the ray in the denser medium (angle of refraction) is less than the angle at which it initially entered the boundary (angle of incidence).





