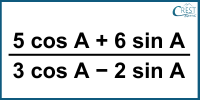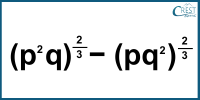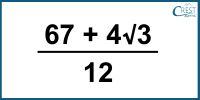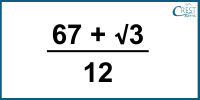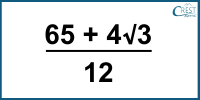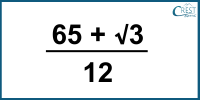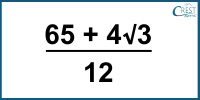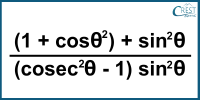Worksheet on Chapter: Trigonometry - Class 10

Solved Questions on Trigonometry

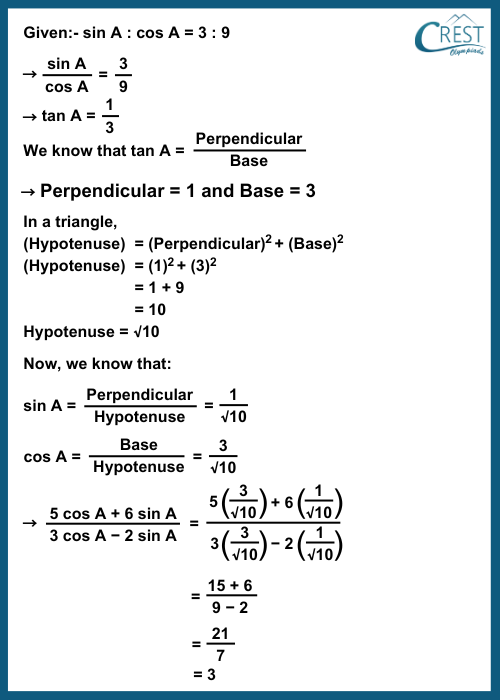
1. If sin A : cos A = 3 : 9, then what is the value of the given trigonometric expression?

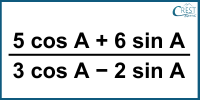
a) 1
b) 2
c) 0
d) 3

Answer: d) 3

Explanation:

2. If cot A + tan A = p and sec A − cos A = q, then what is the value of the given expression?

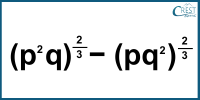
a) 0
b) 1
c) 2
d) − 2

Answer: b) 1

Explanation:

3. If sin A = cos A/√3, then what is the value of sin2 A + sec2 A + sin A sec A + 2 cosec A?

a)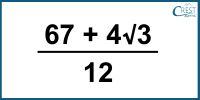
b)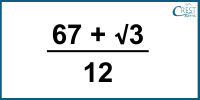
c)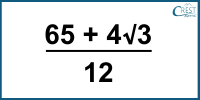
d)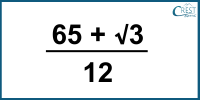

Answer: a)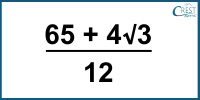

Explanation: 

4. A woman on a cliff observes a boat at an angle of depression of 30° which is approaching the shore to the point immediately beneath the observer with a uniform speed. 18 minutes later, the angle of depression of the boat was found to be 60°. What is the total time taken by the boat to reach the shore?

a) 9 minute
b) 25 minutes
c) 27 minutes
d) 28 minutes

Answer: c) 27 minutes

Explanation: The two positions of the boat at the two instants are P and S and let the speed of the boat be x m/min, h be the height of the cliff and R be the location of the woman.

Thus, the figure is shown as:

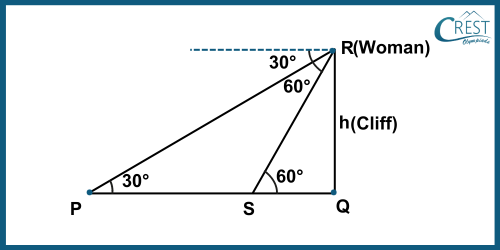
The boat takes 18 minutes to reach point S from point P.

We know that: Distance = Speed × Time                        

Thus, the distance covered by the boat from point P to point S is:

PS = x × 18

PS = 18x

Let the boat take t time to reach the shore, then the distance covered from point S to point Q is:

SQ = xt

Now, apply the trigonometric ratio in the triangle SQR,

tan 60° = ^{Perpendicular}⁄_Base

In the triangle SQR, QR is the perpendicular and SQ is the base whose lengths are QR = h

SQ = xt

→ tan 60° = ^h⁄_xt
→ √3 = ^h⁄_xt
→ h = xt√3 … (1)

Now, apply the trigonometric ratio in the triangle PQR,

tan 30° = ^{Perpendicular}⁄_Base

In the triangle PQR, QR is the perpendicular and PQ is the base whose lengths are QR = h

PQ = PS + SQ = 18x + xt

→ tan 30° = ^h⁄_18x+xt
→ ¹⁄_√3 = ^h⁄_18x+xt
→ h = ^x(18+t)⁄_√3 … (2)

Compare the values h from the equation (1) and equation (2),

→ xt√3 = ^x(18+t)⁄_√3
→ xt√3 × √3 = x(18 + t)
→ 3xt = x(18 + t)
→ 3t = 18 + t
→ 3t − t = 18
→ 2t = 18
→ t = ¹⁸⁄₂

t = 9 mins

Thus, the boat takes 9 minutes to reach the shore from the point S.

∴ Total time taken by the boat to reach the shore Q from point P
= 18 + 9 = 27 minutes

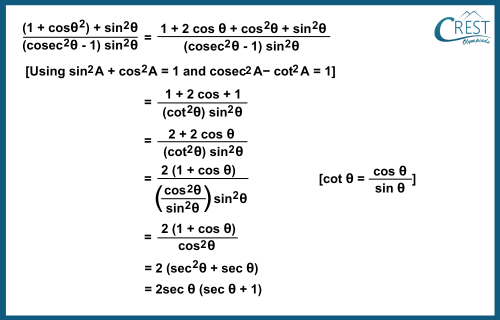
5. Simplify the following trigonometric expression:

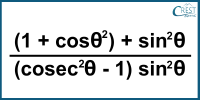
a) 2cos θ (1 + sec θ)
b) sec θ (1 + sec θ)
c) cos θ (1 + sec θ)
d) 2sec θ (1 + sec θ)

Answer: d) 2sec θ (1 + sec θ)

Explanation: